[Econometrics] Consistency in OLS

Consistency : how far the estimator is likely to be from the parameter

 

Let Wn be an estimator of θ based on a sample Y1, Y2,.... Yn of size n. Then Wn is a consistent estimator of

 if for every e > 0,

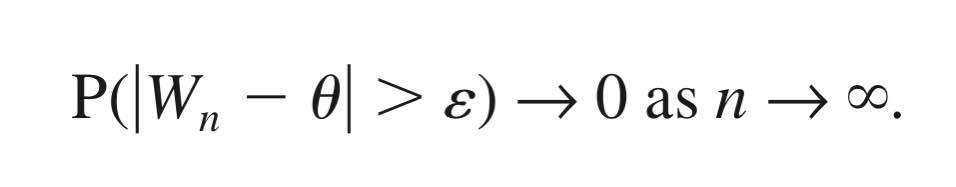
If Wn is not consistent for θ, then we say it is inconsistent.

When Wn is consistent, we also say that θ is the probability limit of Wn ( plim(Wn) = θ ).

Unlike unbiasedness, which is a feature of an estimator for a given sample size, consistency involves the behavior of the sampling distribution of the estimator as the sample size n gets larger. 

 

If an estimator is not consistent then id does not help us to learn about θ, even with an unlimited amount of data. For this reason, consistency is a minimal requirement of an estimator used in statistics or econometrics. When estimators are inconsistent, we can usually find their probability limits, and it will be important to know how far these probability limits are from θ.

 

Unbiased estimators are not necessarily consistent, but those whose variances shrink to zero as the sample size grows are consistent. If Wn is an unbiased estimator of θ and Var(Wn) -> 0 as n -> ∞, then plim(Wn) = θ. Unbiased estimators that use the entire data sample will usually have a variance that shrinks to zero as the sample size grows, thereby being consistent.

 

The good example of a consistent estimator -> Law of Large Numbers

 

In a linear regression model,

even if estimators are not biased, consistency is a minimal requirement for an estimator. For each n, ^β has a probability distribution and if this estimator is consistent, then the distribution of ^β becomes more and more tightly distributed around β as the sample size grows(If ^β is properly estimated).

 

Under assumptions MLR.1 through MLR.4, the OLS estimator ^β is consistent for β.

 

(Need more contents about the consistency in OLS)

 

Resource : Jeffrey M. Woolderfige, "Introductory Econometrics : A Modern Approach 5th edition"